|






| |
Numerical Methods for Ordinary Differential Equation with
Initial Condition:
d y / d t = f ( t , y ) with
y( t = a ) = y_0, a <= t <= b
 |
General Questions
 |
We have existence and uniqueness of the solution when f(t,y)
is continuous and Lipschitz w.r.t its second variable. |
 |
Higher order ODE can always been put in the form above. |
 |
local truncation error, global error |
 |
well-posed, stability, A-stability, consistency, convergence |
 |
ill-conditioned |
 |
stiffness (prefer to use implicit schemes) |
|
 |
Numerical Schemes
 |
Explicit Schemes
 |
Euler's Method (first order) |
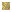 |
Higher Order Taylor Methods
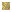 |
give higher order schemes by
calculating higher derivatives of f |
|
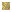 |
Adams-Bashforth Methods
(multi-step)
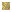 |
give higher order schemes by
profiting from history |
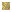 |
need to use one-step method (at
most one order lower) to give starting values |
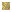 |
ex: AB2 |
|
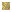 |
Explicit Runge-Kutta Methods (one-step)
 |
give higher order schemes by using
more evaluations of f |
 |
ex: Modified Euler, Midpoint
Method, Heun's Method, RK4 |
|
|
 |
Implicit Schemes
 |
Backward Euler's Method (first
order) |
 |
Implicit Runge-Kutta Methods (one-step) |
 |
Adams-Moulton Methods (multi-step)
 |
give higher order schemes by
profiting from history |
|
 |
Note: need to solve the equation
for y_(k+1) algebraically, iterative method or use predictor-corrector approach
|
|
|
|